Weekly Course Announcements

Welcome Announcement

Week 1

Week 2 Week 3 Week 4

Week 5 Week 6 Week 7 Week 8 Week 9

Week 10 Week 11 Week 12 Week 13 Week 14 Week 15 Week 16

Welcome to MATH 1010: Math for School Teachers

Great Students,

Greetings to everyone.
Welcome to our class. It is great to have you on board.

I am Samuel Chukwuemeka, your instructor for the class. Please do not bite your tongue trying to pronounce my last name 😊
You can call me Mr. Samuel or Mr. C.
Chukwuemeka is a name of Ibo tribe in Nigeria.
Chukwu means GOD; emeka means has done a lot.
So, Chukwuemeka means GOD has done a lot for me.
I have a Bachelor of Engineering degree in Civil Engineering, an Associate in Applied Technology degree in Computer Information Systems, a Master of Education degree in Mathematics Education, and a Master of Science degree in Computer Science. I have taught several mathematics courses at several secondary schools, colleges, and universities for 15 years.
My personal quote is: The Joy of a Teacher is the Success of his Students.
Yes, I mean it. I want you to succeed in your academic profession and I want to be part of that success.

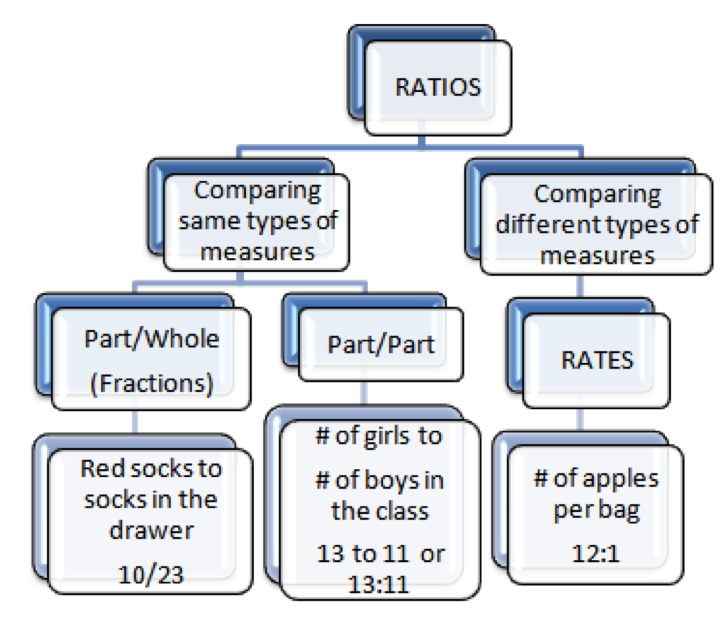
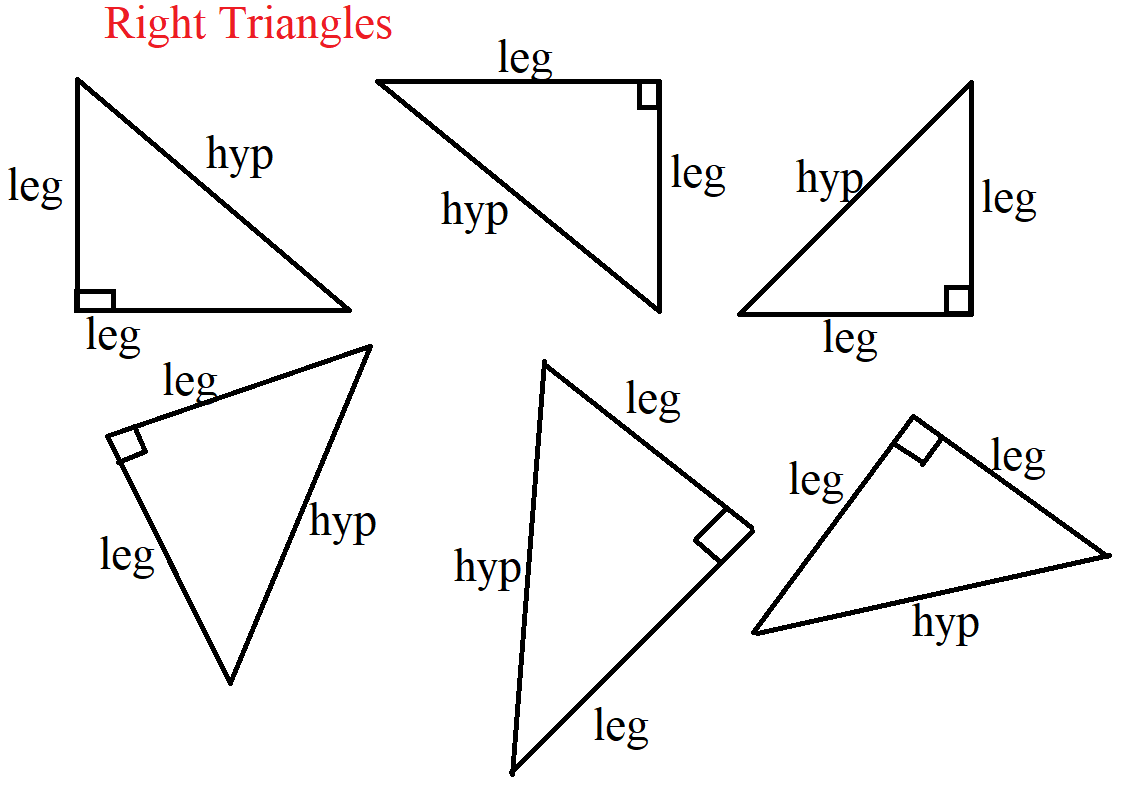
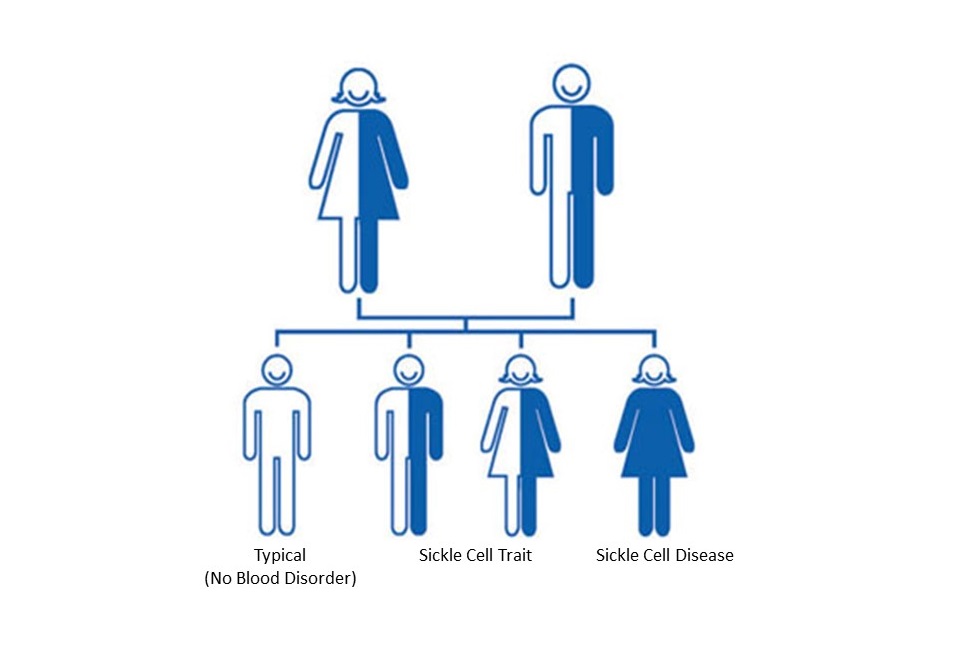
We shall cover these topics: Numbers; Fundamental Principles of Algebra; Measurements and Units; Basic Geometry; Probability; Introductory Statistics; Problem Solving, Reasoning, and Mathematical Communication among others.
We shall apply the knowledge of the topics to real-world problems.
Procrastination is inimical to time. It is important to complete each assessment by the due date. I would plan my time accordingly.

May you please do the following tasks?
Review the course syllabus and all the information in the course.
Weekly Office Hours/Live Sessions will be held on Fridays from 10:30 am – 11:30 am MDT.
Click the invite link (https://wnmu.zoom.us/j/82058513711) and join each week.
There will be a recording of the sessions, so we ask that you do not say or type any information that is insensitive to someone else.
The sessions are optional, however, please make plans to attend.
If the day and time do not suit your schedule, no worries. You can always send an email to me, and we shall communicate accordingly.
Ensure you review all the information for each page and each module. Complete every assessment as applicable. Do not skip.
Feel free to ask questions. I am here to help.
Thank you.

SamDom For Peace

Mathematically Yours,
Samuel Chukwuemeka
Working together for success

Welcome to Week 1: Critical Thinking; Problem Solving; Numbers

Great Students,

Greetings to everyone.
Welcome to Module 1.

One of the skills required for teaching and learning is Critical Thinking skill.
We are encouraged to ask questions or assign tasks/activities that promote critical thinking.
Critical thinking involves the use of our senses to solve problems, or at the mininmum, state ways/directions to solve problems.
In that regard, for this week, we shall solve problems that involve critical thinking.
Then, we shall study the topic of Numbers.
Anything that deals with quantitative data (quantity) involves numbers.

How young are you?
What is your birthday?
How tall are you?
How much is the tuition per credit hour?
How many students are in your program?
How many states are in the United States of America?
What time is it?
...among others

All these questions deal with some amount of quantity. Hence, they are numbers. Do not forget the units. But let us focus on the numbers.
Some numbers are counted, while some numbers are measured.
Some numbers are positive, some numbers are negative. We have only one number that is neutral: neither positive nor negative.
Further, we have nonpositive numbers and nonnegative numbers.

Do you know the difference between positive numbers and nonnegative numbers?
What is the difference between negative numbers and nonpositive numbers?

Welcome to Critical Thinking; Problem Solving; Numbers

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 1 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 2: Fractions and Decimals

Great Students,

Greetings to everyone.
Welcome to Module 2.
We discussed the topic of Numbers in the previous module.
In this module, we shall study some forms of numbers: fractions and decimals.
For this announcement, let us focus on fractions.

Why do we have fractions?
Why do we need fractions?
Why do we study fractions?
Let us review some scenarios where we have used fractions.

Scenario 1
Pizzaiolo: How do you want your pizza after I finish making it: round-cut or square-cut?
Client: There's five of us. So, please divide it into 5 parts either way.

$ \dfrac{part}{whole} = \dfrac{1}{5} \\[5ex] $ This implies that each person gets a part.

Scenario 2
The United States comprises 50 states.
48 states are conterminous states.
49 states are continental states.
(Source: USGS: What constitutes the United States? What are the official definitions? )
This implies that 48 out of the 50 states are conterminous (contiguous) states while 49 states out of the 50 states are continental states.
This implies that:
(a.) The fraction of the U.S states that are conterminous are:

$ \dfrac{part}{whole} = \dfrac{48}{50} = \dfrac{24}{25} \\[5ex] $ (b.) The fraction of the U.S states that are continental are:

$ \dfrac{part}{whole} = \dfrac{49}{50} \\[5ex] $ Scenario 3
Nine out of 10 Nigerians say at least “some” public officials are corrupt.
(Source: Afrobarometer Dispatch No. 187: Page 2: Corruption in Nigeria)

$ \dfrac{part}{whole} = \dfrac{9}{10} \\[5ex] $ ...among many other examples/scenarios.

Welcome to Fractions and Decimals

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 2 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 3: Ratios and Proportions

Great Students,

Greetings to everyone.
Welcome to Module 3.
In the previous module, we discussed the topic of Fractions.
In this module, we shall review Ratios and Proportions.
The pacing guide this week deals with:
Reasoning about Equivalent Fractions...dealing with Proportions.
Reasoning to Compare Fractions...dealing with Ratios.
Sometimes, fractions and ratios are used interchangeably. One of the ways of writing a fraction is to write it as a ratio.
However, it is important to note this difference.

A fraction is the part of a whole, typically written as $\dfrac{part}{whole}$

A ratio is a comparison of two quantities, written as $quantity\;\;unit : quantity\;\;unit$
Notice the colon :
It can also be written as $part: whole$ (another way of writing fraction when the two quantities are the same substance).
The two quantities can be quantities of the same substance or quantities of different substances.
When we compare quantities of the same substances: comparing some amount of that substance to the entire substance, then we are dealing with fractions.
In that sense, we can say that interchangeably use fraction and ratio.
So, we see that fraction is a type of ratio.
This diagram may help with the explanation.

Ratio
(Source: https://matheducators.stackexchange.com/questions/7281/how-to-explain-the-difference-between-the-fraction-a-b-and-the-ratio-a-b)

A proportion is the equality of two ratios.

Welcome to Ratios and Proportions

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 3 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 4: Percents; Exponents; and Scientific Notation

Great Students,

Greetings to everyone.
Welcome to Module 4.
In the previous module, we discussed Ratios and Proportions.
In this module, we shall review one way to convert fractions: Percents.
Then we shall discuss two notations for expressing numbers: Standard Notation and Scientific Notation.
But we cannot discuss the scientific notation without discussing the topic of Exponents.
For this announcement, let us focus on the scientific notation.

Questions for Thought
(1.) What is the speed of light in meters/second (m/s)?
(2.) What is the speed of sound in meters/second (m/s)?
(3.) Have you ever wondered why there must be a lightning before a thunder?
Why do we see the lightning before we hear the thunder?

(4.) Which one (a.) or (b.) do you prefer:
(a.) 299,792,458 m/s
(b.) $3 * 10^8$ m/s
(c.) Which one is expressed in scientific notation?
(d.) Which one is expressed in standard notation?
(e.) Which one includes the base of 10 and an exponent of 8?
(f.) When mathematicians or scientists encounter very large numbers in their work and they need to inform the public, what notation do you think they should use?

Do you understand what I mean so far?
Let us look at another example.
Let us dive to Chemistry/Physics.
(5.) What is the mass of an electron in amu (atomic mass unit)?

(6.) Which one (a.) or (b.) is better to use:
(a.) $5.4858 * 10^{-4}$ amu
(b.) 0.000548579909067 amu
(c.) Which one is expressed in scientific notation?
(d.) Which one is expressed in standard notation?
(e.) Which one includes the base of 10 and an exponent of −4?
(f.) When mathematicians or scientists encounter very small numbers in their work and they need to inform the public, what notation do you think they should use?

Welcome to Scientific Notation; Exponents; and Percents

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 4 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 5: Rates; Proportions; and Percents.

Great Students,

Greetings to everyone.
Welcome to Module 5.
In the previous two modules, we discussed Ratios and Proportions.
In the previous module, we discussed Percents.
In this module, we shall continue our study on Ratios, Proportions, and Percents.

For Ratios:
We shall discuss Rates. Recall that rates and fractions are part of ratios.
A ratio is a comparison of two quantities which could be: quantities of the same substance or quantities of different substances.
When we compare the same types of quantities, we are dealing with fractions. We have already discussed fractions.
When we compare different types of quantities, we are dealing with rates. We shall discuss rates.

For Proportions:
We shall review two main types of proportional relationships: the direct proportional relationship and the inverse proportional relationship.
The more cheeseburgers I eat, the more calories I gain: this is a direct proportional relationship between the number of cheeseburgers and the number of calories.
(Notice I used: number of... to denote some quantity. I did not just say: cheeseburgers and calories. I said: number of cheeseburgers and number of calories.)
The greater the speed of the mode of transportation, the lesser the time it takes to reach the destination: this is an inverse proportional relationship between speed and time.
(Compare the times taken by: an airplane versus a bus to arrive at the same destination.)
We have more types of proportional relationships, but we shall focus on these two types in this module.

For Percents:
We shall solve applied problems on Percent Increase and Percent Decrease.
Example of a Percent Increase: Biden moves to enact 5.2% pay raise for federal employees
(Source: https://www.federaltimes.com/breaking-news/2023/08/31/biden-moves-to-enact-52-pay-raise-for-federal-employees/)

Example of a Percent Decrease: Egg prices drop by nearly 14 percent in May
(Source: https://thehill.com/homenews/4047857-egg-prices-drop-by-nearly-14-percent-in-may/)

Welcome to Rates; Proportions; and Percents.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 5 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 6: Expressions and Equations.

Great Students,

Greetings to everyone.
Welcome to Module 6.
In the previous five modules, we have been dealing with Numbers (constants).
This is generally known as Arithmetic.
In this module, we shall deal with variables and constants.
In other words, we shall begin our study on Algebra.
We shall review the Fundamental Principles of Algebra: Expressions and Equations.

What is an algebraic expression?
What is an algebraic equation?
How do we write from English to Math? In other words, how do we translate between written English and mathematical terminology, concepts, and notation?

Examples: Which of these is: an expression? an equation?
(1.) Nahum bought y children's admission tickets for $2 each x adult's admission tickets for $7 each.
What is the total amount spent by Nahum?

(2.) In August 2009, the United States Senate had a total of 98 Democrats and Republicans.
There were 18 more Democrats than Republicans.
(Source: https://www.thegreenpapers.com/)
How many Democrats were there?
How many Independents were there?

(3.) Can you calculate my age in the year 2008?
Three hundred reduced by three times my age is one hundred and ninety two.
What was my age?

Welcome to Expressions and Equations.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 6 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 7: Relations and Functions; Lines and Angles.

Great Students,

Greetings to everyone.
Welcome to Module 7.
Last week, we began our study on Algebra.
We reviewed the Fundamental Principles of Algebra: Expressions and Equations.
This week, we shall extend our study by looking into functions. Specifically, we shall study linear functions.
Are there any differences between an expression, an equation, and a function?

$ 2x + 3 ...linear\;\;expression \\[3ex] 2x + 3 = -5 ...linear\;\;equation \\[3ex] f(x) = 2x + 3 ...linear\;\;function \\[3ex] Also:\;\;2x + 3 ...linear\;\;function \\[3ex] $ So, as we see; functions can take the form of expressions or equations.
But we cannot discuss functions without discussing relations because a function is first and foremost a relation.
One of the ways to represent relations is by using a Graph.
This leads us to Lines.
Intersection of two lines creates an Angle.
We shall review lines and angles as well.
For this announcement, let us focus on relations and functions.

Before I immigrated to the United States, I looked like this:
SamDom

Then, I came here, ate a lot of cheeseburgers, gained a lot of weight, and now look like this:
SamDom For Peace
(Please don't laugh at me) 😊

So we have two variables: weight and the number of cheeseburgers.
Which one depends on the other?
In other words:
(1.) Which of the variables is the dependent variable?
(2.) Which one is the independent variable?
(Hint: Does my weight depend on the number of cheeseburgers I eat, or does the number of cheeseburgers I eat depend on my weight?)

Questions for Thought: First Set
(1.) Did you notice any relationship?
What is the relationship?
Any input-output relationship?
Which variable is the input?
Which variable is the output?
(2.) Can you express that relationship as a Set of ordered pairs (set of points)?
(3.) Can you express that relationship as a Function Rule (Equation)?
(4.) Can you express that relationship as a Table of Values?
(5.) Can you express that relationship as a Graph?

This example is a two-variable relationship where the weight is the dependent variable and the number of cheeseburgers is the independent variable.
In the example, we say that the weight, w is a function of the number of cheeseburgers, c
We can write it as:
w = f(c)
Let us make some interdisciplinary connections:

Bring it to Algebra and Calculus
y is the dependent variable
x is the independent variable

Bring it to Statistics
y is the response variable
x is the predictor or explanatory variable

Bring it to Philosophy (Cause-Effect Relationship)
y is the effect
x is the cause

Bring it to Economics/Business (Input-Output Relationship)
y is the output
x is the input

Bring it to Psychology/Human Behavior/Sociology
y is the consequence
x is the action

So, for any input, there is at least an output. This is defined as a Relation.
Welcome to Relations.

Notice the word, at least in the definition of a Relation.
At least one output means one or more output.
But, is it possible for an input to have more than one output?
Is it possible for a WNMU student to have more than one Student ID?
Is it possible for an American to have more than one SSN?
Is it possible for a student to make more than one grade on the same test?

This leads us to these concepts:
Function
One-to-one Function (Injective Function)
Onto Function (Surjective Function)
Bijective Function

Questions for Thought: Second Set
Determine if these scenarios represent a relation, function, one-to-one function, onto function, surjective function.
Write all that is applicable.
(1.) A WNMU student has only one Student ID.
(2.) A WNMU student has more than one Student ID.
(3.) Two WNMU Students have the same grade on a Math quiz.
(4.) A WNMU student has two different grades on a Math quiz.
(5.) Every WNMU student was born by a woman.

Welcome to Relations and Functions; Lines and Angles.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 7 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 8: Mensuration.

Great Students,

Greetings to everyone.
Welcome to Module 8.
In the previous module, we started our introduction to geometry by discussing Lines and Angles.
In this module, we shall continue our study on Angles.
Then, we shall extend our study of objects that have lines, angles, and surfaces...known as geometric figures or shapes.
This is an introduction to Mensuration: the branch of mathematics that deals with the measurements of geometric figures and their parameters.
Specifically, we shall analyze the properties of these geometric figures including Circles, Spheres, Triangles, Quadrilaterals, and other Polygons.

Where are you right now?
Hmmmm...Mr. C, are you trying to monitor me? 😊
No, I'm not monitoring you. I just want to make a connection to what we are about to discuss this week.
What do you see? Do you see any geometric figure/shape? If you are in the classroom, library, church, or your room, there is a high probability that you will see at least one geometric shape.
What geometric figure do you see?
Is it regular or irregular?
Is it 2-dimensional or 3-dimensional?
Can you list and analyze the properties of the figure?

Welcome to Mensuration.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 8 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 9: Mensuration (Continued).

Great Students,

Greetings to everyone.
Welcome to Module 9.
In the module, we shall continue our study on the Mensuration of basic shapes including circles, triangles, quadrilaterals, and other polygons.
Last week, we reviewed the properties of these shapes.
This week, we shall calculate the mensuration of these shapes.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 9 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 10: Measurements and Units.

Great Students,

Greetings to everyone.
Welcome to Module 10.
In the previous module, we calculated the mensuration (measurements of parameters) of basic shapes including circles, triangles, quadrilaterals, and other polygons.
In this module, we shall discuss two important systems of measurements and units.

Look around you.
Do you find any container such as containers of food, drinks, soap, paint, lotion?
Look at the entire container. Do you see any measurements? units?
How many measurements (values) do you see?
How many units do you see?
Most likely, you saw at least two different measurements and two different units?

Questions for Thought
(1.) Which unit is the United States/Customary unit?
(2.) Which unit is the International/Metric unit?
(3.) Why are there at least two different measurements and two different units?
(Hint: If an American company that produces food in containers wants to market it to Mexico, is it not appropriate for the company to use the unit that Mexico uses for the measurement of food?
Similarly, If an Mexican company that produces food in containers wants to market it to America, is it not appropriate for the company to use the unit that America uses for the measurement of food?)
(4.) Is it possible to have errors in measurements?
What are the errors in measurements?
If two or more people measure the length of a desk, would they obtain the same measurement to the: nearest integer, tenth, hundredth, ..., unit?
...and so on and so forth.

Welcome to Measurements and Units.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 10 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 11: Mensuration (Continued).

Great Students,

Greetings to everyone.
Welcome to Module 11.
In the previous module, we deviated a bit to discuss the systems of measurements and units for basic shapes including circles, triangles, quadrilaterals, and other polygons.
In this module, we shall continue our study on the Mensuration of these shapes.
Specifically, we shall determine the areas of the shapes.
Further, we shall solve applied problems involving the areas of the shapes.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 11 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 12: Mensuration (Continued).

Great Students,

Greetings to everyone.
Welcome to Module 12.

In the previous module, we determined the areas of basic shapes including circles, triangles, quadrilaterals, and other polygons.
In this module, we shall continue our study on the Mensuration of these shapes.
Specifically, we shall determine the perimeters of the shapes.
Then, we shall discuss the relationship between the areas and the perimeters of the shapes.
Further, we shall discuss the Pythagorean Theorem.
Let us focus on the Pythagorean Theorem in this announcement.
The theorem deals with the relationship between the sides of a right triangle.
Let us consider this activity that you can do with your students.

Classroom Activity
Teacher: Hosea, please come.
Take that chair and mark as your starting point.
Walk four steps in a horizontal line.
Then, turn around at an angle of 90°
In other words, rotate left 90°
Walk three steps in a vertical line.
Then, turn towards me to face me.
If I asked you to come to me, how many steps would you walk to reach me?
Student: About 5 steps I guess...
Ask your students and note their responses.
Ask them to give reasons for their answers.
Teacher: About...indicates you are not so sure?
Well, it is 5 steps.
That is correct.
But, how did you get 5 steps?
Student: Mr. C, it's just a guess...a correct guess.
I'll just walk towards you 😊
Teacher: Let's find out.
Welcome to the Pythagorean Theorem

It states that:
In a right triangle, the square of the length of the hypotenuse is the sum of the squares of the other two sides.
Let the: long side = hypotenuse = hyp
middle side = middle = leg
short side = short = leg

$hyp^2 = short^2 + middle^2$

OR

In a right triangle, the square of the length of the hypotenuse is the sum of the squares of the other two sides.
$hyp^2 = leg^2 + leg^2$

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 12 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 13: Mensuration (Continued).

Great Students,

Greetings to everyone.
Welcome to Module 13.

In the previous module, we determined the perimeters of the shapes.
We discussed the relationship between the areas and the perimeters of the shapes, and the Pythagorean Theorem.
In this module, we shall continue our study of Mensuration by discussing solid shapes.
We have been mainly discussing two-dimensional shapes.
Now, we have to discuss three-dimensional shapes also known as solids or solid shapes.
They are three-dimensional shapes because they have three dimensions: length, width, and height.
Examples include: cube, cuboid, cone, cylinder, sphere, prism, and pyramid.
We shall determine the mensuration of these solid shapes.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 13 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 14: Introductory Statistics and Descriptive Statistics.

Great Students,

Greetings to everyone.
Welcome to Module 14.

In this module, we shall discuss Introductory Statistics.
Then, we shall discuss Descriptive Statistics.
Let us begin with Introductory Statistics.

Questions for Thought:
(1.) Did you register for this course?
(Of course...Mr. C, what kind of question is that? Why did I have access to the Canvas course if I did not register for it?)
Are you familiar with any of these?
Name:
Date of Birth:
Age:
Address:
...among others
All of the information you submitted when you enrolled in WNMU or any other academic institution is known as Data.
You provided these data to WNMU.
Have you ever wondered: what does WNMU do with these data?

(2.) Some of you are on social media platforms: YouTube, Facebook, Twitter, TikTok, Instagram, Snapchat among others.
You gave these platforms your data when you registered for their services.
Have you asked yourself: what do these platforms do with my data?

(3.) In your daily conversation/interaction/communication with people, have you discussed: race, gender, color, age, religion, temperature, height, weight, number of people, number of something, etc.? You have discussed data.

Statistics is all about data:
Data Collection: How do you collect data?
Data Organization: What do you do with the raw data? Is it not better to organize it before you use it?
Data Presentation: What are the several tools to present this data so it makes meaning to everyone?
Data Analysis: How do we analyze this data? What evidence/information/results can we get by analyzing the data? How do we use the results of the analysis?
Data Interpretation: After analyzing the data, do we need to use our results to make the right decision and the right conclusion?

Welcome to Introductory Statistics.

Do you know the first part of data analysis?
When we collect data, organize it, and present it, what else can we do with the data?...Analyze it and note the results of the analysis.
We shall descriptively analyze data into:
Measures of Center
Measures of Spread
Measures of Position and
Measures of Shape.

Focusing on the Measures of Center; let us begin with this story.
Yes, I tell stories too 😊

It was a Saturday
A family of the Dad, Mom, Daughter, Son
The two children are in middle school.
The Dad and Mom were reviewing the notes of their children
The children were reading books.
All of them were in the living room at home.

Dad: Elijah
Son: Yes, Dad
Dad: Esther
Daughter: Yes, Dad
Dad: A philanthropist wanted to buy shoes for the motherless and fatherless children at an orphanage.
There were 300 children at the orphanage.
He arrived at the orphanage and asked for a shoe size.
What shoe size should the director recommend?
Son: He asked for just a shoe size...
Rather than shoe sizes?
... continue with the rest of the story

Welcome to Descriptive Statistics.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 14 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success

Welcome to Week 15: Complete all Assignments.

Great Students,

Greetings to everyone.
Welcome to Module 15.

HAPPY THANKSGIVING.
Please complete all outstanding assignments.

Samuel Chukwuemeka
Working together for success

Welcome to Week 16: Probability

Great Students,

Greetings to everyone.
Welcome to Module 16.

In the previous module, we went for Thanksgiving Break. I asked you to complete all outstanding assignments.
In the previous two modules, we discussed the topic of Introductory Statistics.
Remember that one of the reasons for analyzing data is to make a good decision and a good conclusion.

In this final module, we shall discuss the topic of Probability.
What is the probability of an event?
Before we discuss the probability of an event, we need to explain the meaning of an event.
What is an event?
How many types of events do we have?
What are the laws (rules) of probability?
How can I apply the topic of Probability in life?
That is our focus in this announcement. Please review the topic of Probability in your textbook (eText) and the resources I provided for you in the Pacing Guide.
For this announcement, let us discuss one important application of probability: Probability in Biology.

If you are not yet married, may I advise you to review this application of probability and consider it?
I am not trying to influence you in any way.
I am only asking you to consider this application of probability.
How can we make a good decision and a good conclusion? (Recall the reasons for Data Analysis in Statistics)
Well, we can apply that knowledge in this topic of Probability.

Probability in Biology:
People who inherit one sickle cell gene and one normal gene have sickle cell trait (SCT).
People with SCT usually do not have any of the symptoms of sickle cell disease (SCD), but they can pass the trait on to their children.
[What is Sickle Cell Trait? (https://www.cdc.gov/ncbddd/sicklecell/traits.html)]
Sickle cell disease (SCD) is a group of inherited red blood cell disorders.
Red blood cells contain hemoglobin, a protein that carries oxygen.
Healthy red blood cells are round, and they move through small blood vessels to carry oxygen to all parts of the body.
In someone who has SCD, the hemoglobin is abnormal, which causes the red blood cells to become hard and sticky and look like a C-shaped farm tool called a sickle.
The sickle cells die early, which causes a constant shortage of red blood cells.
Also, when they travel through small blood vessels, they get stuck and clog the blood flow. This can cause pain and other serious complications (health problems) such as infection, acute chest syndrome and stroke.
[What is Sickle Cell Disease? (https://www.cdc.gov/ncbddd/sicklecell/facts.html)]

Probability Application: Probability in Biology

A man and a woman wants to marry.
Both have the sickle cell trait (SCT).
They do not know about Probability in Biology, but they saw this diagram at the CDC’s website (Centers for Disease Control and Prevention's website).
They are aware that you took a Statistics class with Mr. C and that you might help explain the diagram.

(a.) Using a Tree Diagram and/or a Punnett Square, explain the diagram to the man and his fiancée.
Include the concept of Probability in your explanations. Assume they intend to have four children.

(b.) Should they get married or not? Advise them.
( To see my advice, please review the Applications of Probability )

Welcome to Probability.

May you please:
(1.) Review the Overview and Objectives.
(2.) Review the Readings/Assessments.
(3.) Complete the assessments initially due this week.
(4.) Participate in the Week 16 Discussion.
(5.) Attend the Live Sessions/Office Hours for this week.

Should you have any questions, please ask. I am here to help.
Thank you.

Samuel Chukwuemeka
Working together for success