Solved Examples: Angles



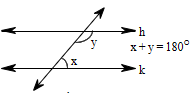
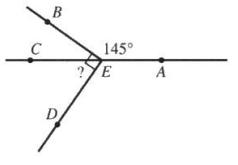
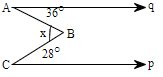
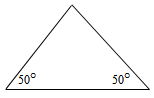
Samuel Dominic Chukwuemeka (SamDom For Peace) Formulas:
(1.) Geometry Formulas
(2.) Mensuration Formulas

For ACT Students
The ACT is a timed exam...60 questions for 60 minutes
This implies that you have to solve each question in one minute.
Some questions will typically take less than a minute a solve.
Some questions will typically take more than a minute to solve.
The goal is to maximize your time. You use the time saved on those questions you solved in less than a minute, to solve the questions that will take more than a minute.
So, you should try to solve each question correctly and timely.
So, it is not just solving a question correctly, but solving it correctly on time.
Please ensure you attempt all ACT questions.
There is no negative penalty for any wrong answer.

For JAMB and CMAT Students
Calculators are not allowed. So, the questions are solved in a way that does not require a calculator.

For NSC Students
For the Questions:
Any space included in a number indicates a comma used to separate digits...separating multiples of three digits from behind.
Any comma included in a number indicates a decimal point.
For the Solutions:
Decimals are used appropriately rather than commas
Commas are used to separate digits appropriately.

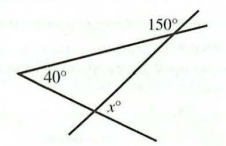
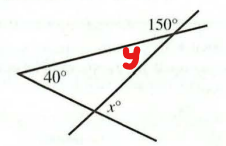
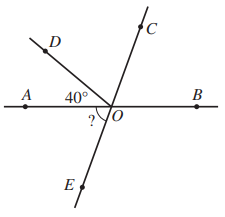
Solve all questions
Give reasons accordingly.
Show all work
(1.) A student made several mathematical statements/sentences or asked questions regarding angles.
As a teacher, how would you respond?

(a.) Why​ don't we use the term​ equal angles instead of​ congruent angles?

(b.) If one angle of a triangle is​ obtuse, another angle can also be​ obtuse.

(c.) A triangle has two right​ angles.

(d.) If one angle of a triangle is​ acute, the other two angles also be​ acute.

(e.) If a triangle has one acute​ angle, then the triangle is​ an acute triangle.

(f.) $\overline{AB}$ ≠ $\overline{BA}$ because:
$\overline{AB}$ starts at A and ends at​ B, and $\overline{BA}$ starts at B and ends at A.

(g.) There can only be 360 different rays emanating from a point since there are only 360 ° in a circle.

(h.) It is actually impossible to measure an​ angle, since each angle is the union of two rays that extend infinitely and therefore continue forever.

(i.) Can two adjacent angles be vertical​ angles?


(a.) Since an angle is a set of points determined by two rays with the same​ endpoint, to say that two angles are equal implies that the two sets of points determining the angles are equal.
The only way this can happen is if the two angles are actually the same angle.

(b.) The sum of the angles of a triangle is 180°.
The sum of two obtuse angles is more than 180°. So, this is not possible.

(c.) The sum of the angles of a triangle is 180°.
The sum of two right angles is 180°.
The sum of two right angles and the third angle is more than 180°. So, this is not possible.

(d.) This is possible because the three angles that are less than 90° can sum to 180°

(e.) An acute triangle is a triangle with three acute angles.
If a triangle has one acute angle, the triangle is not necessarily an acute triangle because the triangle can have one acute angle and a right angle or an obtuse angle.

(f.) Please note that:
(1st.) Lines and line segments do not have a​ direction, and any two points uniquely determine both lines and line segments.
(2nd.) Lines​ do not have endpoints. Line segments do.

(g.) As a degree can be subdivided​ infinitely, there are an infinite number of points that lie on a circle that can be used for the second point of a ray.

(h.) Angle measurements do not relate to length.
Angles are measured by the amount of​ "opening" between their sides (the amount of turn between the sides) around their vertex.

(i.) ​No, two adjacent angles cannot be vertical​ angles.
Adjacent angles share a common side while vertical angles do not.

(2.)

(3.) ACT In the figure below, $\overline{XW}$ intersects $\overline{VZ}$ at Y, the measure of $\angle XYV$ is (3x + 5)°, and the measure of $\angle ZYW$ is (4x − 6)°
What is the measure of ∠XYZ?

Number 3

F. 83°
G. 97°
H. 104°
J. 142°
K. 169°


$ 4x - 6 = 3x + 5 ...vertical\;\angle s\;\;are\;\;congruent \\[3ex] 4x - 3x = 5 + 6 \\[3ex] x = 11 \\[3ex] \angle XYZ + (3x + 5) = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] \angle XYZ + [3(11) + 5] = 180 \\[3ex] \angle XYZ + 38 = 180 \\[3ex] \angle XYZ = 180 - 38 \\[3ex] \angle XYZ = 142^\circ $
(4.)


(5.) In the diagram below:

Number 5

Why is h ∥ k?


Construction: Label angle p

$ \angle x + \angle y = 180^\circ ...Given \\[3ex] \angle x = 180 - y \\[5ex] \angle p + \angle y = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] \angle p = 180 - y \\[5ex] \implies \angle x = \angle p = 180 - y \\[3ex] \implies Also:\;\; \angle x = \angle p ...corresponding\;\;\angle s\;\;are\;\;congruent \\[3ex] Hence:\;\; \overleftrightarrow{h} \;\;\parallel\;\; \overleftrightarrow{k} \\[3ex] $ Theorem: If the corresponding angles of two lines are equal, then the lines must be parallel.
(6.)

(7.) ACT In the figure below, E is on $\overline{CA}$, and the measures of $\angle BED$ and $\angle AEB$ are 90° and 145° respectively.
If it can be determined, what is the measure of $\angle CED$?

Number 7

A. 35°
B. 45°
C. 55°
D. 80°
E. Cannot be determined from the given information


$ \angle CEB + \angle AEB = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] \angle CEB + 145 = 180 \\[3ex] \angle CEB = 180 - 145 \\[3ex] \angle CEB = 35^\circ \\[5ex] \angle CED + \angle CEB = \angle BED ...diagram \\[3ex] \angle CED + 35 = 90 \\[3ex] \angle CED = 90 - 35 \\[3ex] \angle CED = 55^\circ $
(8.)


(9.) Determine the value of x or the missing value in these diagrams.

(a.) Number 9a
s ∥ r

(b.) Number 9b
q ∥ p

(c.) Number 9c


(d.) Number 9d



(a.) Construction:
Extend line segment AB
Label ∠p and ∠k

Number 9a

$ p + 147 = 180^\circ ... consecutive\;\;interior\;\angle s\;\;are\;\;supplementary \\[3ex] p = 180 - 147 \\[3ex] p = 33^\circ \\[5ex] k + 120 = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] k = 180 - 120 \\[3ex] k = 60^\circ \\[5ex] x = p + k ...exterior\;\angle \;\;of\;\;a\;\;\triangle = sum\;\;of\;\;two\;\;interior\;\;opposite\;\;\angle s \\[3ex] x = 33 + 60 \\[3ex] x = 93^\circ \\[3ex] $ (b.) Construction:
Extend line segment CB
Label ∠p

Number 9b

$ p = 28^\circ ... alternate\;\;interior\;\angle s\;\;are\;\;congruent \\[3ex] x = p + 36 ...exterior\;\angle \;\;of\;\;a\;\;\triangle = sum\;\;of\;\;two\;\;interior\;\;opposite\;\;\angle s \\[3ex] x = 28 + 36 \\[3ex] x = 64^\circ \\[3ex] $ (c.) Let the missing angle = x

$ x + 50 + 50 = 180^\circ ...sum\;\;of\;\;\angle s\;\;of\;\;a\;\;\triangle \\[3ex] x + 100 = 180 \\[3ex] x = 180 - 100 \\[3ex] x = 80^\circ \\[3ex] $ (d.) Let the missing angle = x

$ x + 90 + 45 = 180^\circ ...sum\;\;of\;\;\angle s\;\;of\;\;a\;\;\triangle \\[3ex] x + 135 = 180 \\[3ex] x = 180 - 135 \\[3ex] x = 45^\circ \\[3ex] $
(10.)


(11.) ACT In the figure below, $\overline{AB}$ is congruent to $\overline{BC}$, and $\overline{AE}$ intersects $\overline{BF}$ at C.
What is the measure of $\angle B$?

Number 11

A. 14°
B. 38°
C. 76°
D. 104°
E. 142°


$ \angle BCA = \angle ECF = 38^\circ ...vertical\;\angle s\;\;are\;\;congruent \\[3ex] \angle BAC = \angle BCA = 38^\circ ...\overline{AB} = \overline{BC} ...base\;\;\angle s\;\;of\;\;isosceles\;\; \triangle ABC \\[3ex] \angle B + \angle BAC + \angle BCA = 180^\circ ...sum\;\;of\;\;\angle s\;\;in\;\;\triangle ABC \\[3ex] \angle B + 38 + 38 = 180 \\[3ex] \angle B + 76 = 180 \\[3ex] \angle B = 180 - 76 \\[3ex] \angle B = 104^\circ $
(12.)

(13.) Answer the following questions.
(a.) If two angles of a triangle are​ complementary, what is the measure of the third​ angle?

(b.) Determine the measure of an angle whose measure is 14 times that of its complement.

(c.) What is the measure of the complement of the supplement of a 131° ​angle?

(d.) If three lines all meet in a single​ point, how many pairs of vertical angles are​ formed?


(a.) Two angles are complementary if the sum of the two angles is a right angle

$ third\;\angle + two\;\;\angle s = 180^\circ ...sum\;\;of\;\;\angle s\;\;of\;\;a\;\;\triangle \\[3ex] third\;\angle + 90 = 180 \\[3ex] third\;\angle = 180 - 90 \\[3ex] third\;\angle = 90^\circ \\[3ex] $ (b.) Let the angle = x
Complement of the angle = 90 − x

$ x = 14(90 - x) \\[3ex] x = 1260 - 14x \\[3ex] x + 14x = 1260 \\[3ex] 15x = 1260 \\[3ex] x = \dfrac{1260}{15} \\[5ex] x = 84^\circ \\[3ex] $ (c.) ∠ = 131°
Supplement of 131° = 180 - 131 = 49°
Complement of 49° = 90 - 49 = 41°

(d.) Let us represent the information using a diagram
Each pair of vertical lines is labelled with the same color.

Number 13d

$ \underline{Vertical\;\angle s} \\[3ex] \alpha = \alpha \\[3ex] \beta = \beta \\[3ex] \theta = \theta \\[3ex] $ If three lines all meet in a single​ point, 3 pairs of vertical angles are formed.
(14.) Determine how many acute angles are determined in this figure.
List them.

Number 14


An acute angle is an angle less than 90°
The acute angles in the figure are:
$\angle AOB$     $\angle BOC$     $\angle COD$
$\angle AOC$     $\angle BOD$     $\angle COE$
$\angle AOD$     $\angle BOE$     $\angle COF$
$\angle AOE$     $\angle BOF$

$\angle DOE$     $\angle EOF$
$\angle DOF$
(15.) ACT In the figure below, line m is perpendicular to line n, and line p is parallel to line q
Lines m, n, and p intersect at a single point.
Some angle measures are given.
What is the value of x?

Number 15

$ F.\;\; 32 \\[3ex] G.\;\; 58 \\[3ex] H.\;\; 122 \\[3ex] J.\;\; 148 \\[3ex] K.\;\; 158 \\[3ex] $

We can solve the question using at least two approaches.
Use any approach you prefer.

1st Approach:
Number 15-1st

$ p \parallel q \\[3ex] n\;\;is\;\;the\;\;transversal \\[3ex] k = 32^\circ ...corresponding\;\angle s\;\;are\;\;congruent \\[3ex] x + k = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] x + 32 = 180 \\[3ex] x = 180 - 32 \\[3ex] x = 148^\circ \\[3ex] $ 2nd Approach:
Number 15-2nd

$ p \parallel q \\[3ex] n\;\;is\;\;the\;\;transversal \\[3ex] p = 32^\circ ...vertical\;\angle s\;\;are\;\;congruent \\[3ex] h = p = 32^\circ ... alternate\;\;interior\;\angle s\;\;are\;\;congruent \\[3ex] x + h = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] x + 32 = 180 \\[3ex] x = 180 - 32 \\[3ex] x = 148^\circ $
(16.)

(17.)

(18.)


(19.) ACT Three line segments intersect as shown in the figure below, forming angles with measures of 150°, 40°, and x°, respectively.
What is the value of x?

Number 19

$ F.\;\; 95 \\[3ex] G.\;\; 85 \\[3ex] H.\;\; 80 \\[3ex] J.\;\; 75 \\[3ex] K.\;\; 70 \\[3ex] $

Number 19

$ y + 150^\circ = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] y = 180 - 150 \\[3ex] y = 30^\circ \\[5ex] x = 40 + y ...exterior\;\angle \;\;of\;\;a\;\;\triangle = sum\;\;of\;\;two\;\;interior\;\;opposite\;\;\angle s \\[3ex] x = 40 + 30 \\[3ex] x = 70^\circ $
(20.)





Top




(21.) ACT In the figure below, $\overleftrightarrow{AB}$ and $\overleftrightarrow{CE}$ intersect at O, $\overrightarrow{OC}$ bisects $\angle BOD$, and the measure of $\angle AOD$ is 40°.
What is the measure of $\angle AOE$ ?

Number 21

$ F.\;\; 40^\circ \\[3ex] G.\;\; 50^\circ \\[3ex] H.\;\; 60^\circ \\[3ex] J.\;\; 70^\circ \\[3ex] K.\;\; 80^\circ \\[3ex] $

$\overrightarrow{OC}$ bisects $\angle BOD$
⇒
$\angle BOC = \angle COD = x$

$ 40 + \angle COD + \angle BOC = 180^\circ ...\angle s\;\;on\;\;a\;\;straight\;\;line \\[3ex] 40 + x + x = 180 \\[3ex] 2x = 180 - 40 \\[3ex] 2x = 140 \\[3ex] x = \dfrac{140}{2} \\[5ex] x = 70^\circ \\[3ex] \angle AOE = x = 70^\circ ...vertical\;\angle s\;\;are\;\;congruent $
(22.)


(23.)


(24.)

(25.)

(26.)

(27.)

(28.)


(29.)

(30.)

(31.)


(32.)


(33.)

(34.)

(35.)

(36.)

(37.)

(38.)

(39.)


(40.)






Top




(41.)


(42.)

(43.)

(44.)